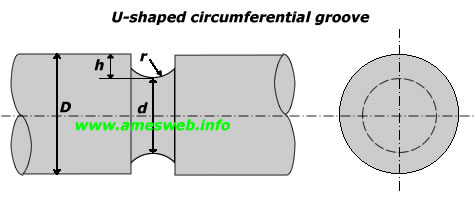
U-SHAPED CIRCUMFERENTIAL GROOVE ON A SHAFT

U-shaped circumferential groove on a shaft. Theoretical stress concentration factors (K_t) calculator for tension, bending and torsion loads.

INPUT PARAMETERS
Parameter	Value
Diameter of larger shaft section [D]		mm cm m inch ft
Diameter of smaller shaft section [d]
Radius [r]
Tension force [P]		N kN lbf
Bending moment [M]		N*m lbf*in lbf*ft
Torque [T]

Note:Use dot "." as decimal separator.

Please Wait... 

RESULTS
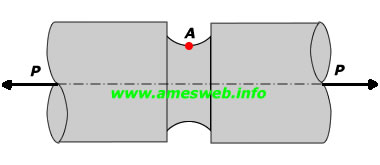
LOADING TYPE - TENSION
Parameter	Value
Stress concentration factor [Kt] *	---	---
Nominal tension stress at shaft [σnom ] o	---	MPa psi ksi
Maximum tension stress due to tension load (at Point-A) [σmax ]	---
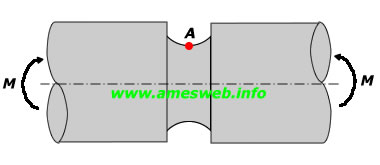
LOADING TYPE - BENDING
Parameter	Value
Stress concentration factor [Kt] *	---	---
Nominal tension stress at shaft [σnom ] +	---	MPa psi ksi
Maximum tension stress due to bending (at Point-A) [σmax ]	---
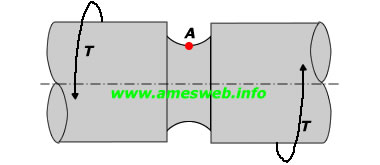
LOADING TYPE - TORSION
Parameter	Value
Stress concentration factor [Kt] **	---	---
Nominal shear stress at shaft [τnom ] x	---	MPa psi ksi
Maximum shear stress due to torsion (at Point-A) [τmax ]	---

Note 1: Maximum stress is occurred at point A.

Note 2: * Geometry rises σ_nom by a factor of K_t .  (K_t = σ_nom/σ_max)

Note 3: ** Geometry rises τ_nom by a factor of K_t . (K_t = τ_nom/τ_max)

Note 4: ^o σ_nom = 4P/(πd²) (Nominal tension stress occurred due to tension load)

Note 5: ⁺ σ_nom = 32M/(πd³) (Nominal tension stress occurred due to bending)

Note 6: ^x   τ_nom = 16T/(πd³) (Nominal shear stress occurred due to torsion)

Definitions:

Stress Concentration Factor: Dimensional changes and discontinuities of a member in a loaded structure causes variations of stress and high stresses concentrate near these dimensional changes. This situation of high stresses near dimensional changes and discontinuities of a member (holes, sharp corners, cracks etc.) is called stress concentration. The ratio of peak stress near stress riser to average stress over the member is called stress concentration factor.

K_t: Theoretical stress concentration factor in elastic range = (σ_max/σ_nom)

Formulas:

Tension
	0.1 ≤ h/r ≤ 2.0	2.0 ≤ h/r ≤ 50.0
C1	$$0.89+2.208\sqrt { h/r } -0.094h/r$$	$$1.037+1.967\sqrt { h/r } +0.002h/r$$
C2	$$-0.923-6.678\sqrt { h/r } +1.638h/r$$	$$-2.679-2.980\sqrt { h/r } -0.053h/r$$
C3	$$2.893+6.448\sqrt { h/r } -2.516h/r$$	$$3.090+2.124\sqrt { h/r } +0.165h/r$$
C4	$$-1.912-1.944\sqrt { h/r } +0.963h/r$$	$$-0.424-1.153\sqrt { h/r } -0.106h/r$$
Kt=C1+C2(2h/D)+C3(2h/D)2+C4(2h/D)3
σnom=4P/πd2
σmax=σA=Ktσnom
Bending
	0.25 ≤ h/r ≤ 2.0	2.0 ≤ h/r ≤ 50.0
C1	$$0.594+2.958\sqrt { h/r } -0.520h/r$$	$$0.965+1.926\sqrt { h/r }$$
C2	$$0.422-10.545\sqrt { h/r } +2.692h/r$$	$$-2.773-4.414\sqrt { h/r } -0.017h/r$$
C3	$$0.501+14.375\sqrt { h/r } -4.486h/r$$	$$4.785+4.681\sqrt { h/r } +0.096h/r$$
C4	$$-0.613-6.573\sqrt { h/r } +2.177h/r$$	$$-1.995-2.241\sqrt { h/r } -0.074h/r$$
Kt=C1+C2(2h/D)+C3(2h/D)2+C4(2h/D)3
σnom=32M/πd3
σmax=σA=Ktσnom
Torsion
	0.25 ≤ h/r ≤ 2.0	2.0 ≤ h/r ≤ 50.0
C1	$$0.966+1.056\sqrt { h/r } -0.022h/r$$	$$1.089+0.924\sqrt { h/r } +0.018h/r$$
C2	$$-0.192 - 4.037\sqrt { h/r } +0.674h/r$$	$$-1.504 - 2.141\sqrt { h/r } -0.047h/r$$
C3	$$0.808 +5.321\sqrt { h/r } -1.231h/r$$	$$2.486 +2.289\sqrt { h/r } +0.091h/r$$
C4	$$-0.567-2.364\sqrt { h/r } +0.566h/r$$	$$-1.056 -1.104\sqrt { h/r } -0.059h/r$$
Kt=C1+C2(2h/D)+C3(2h/D)2+C4(2h/D)3
τnom=16T/πd3
τmax=τA=Ktτnom

Reference:

• Pilkey, W. D..(2005). Formulas for Stress, Strain, and Structural Matrices Formulas for Stress, Strain, and Structural Matrices .2nd Edition John Wiley & Sons